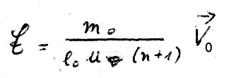Thus, we only have quantum rules because there is no continuous spectrum of elementary charges. Consequently, the root of all quantum rules lies in the fact that we find only one specific elementary charge in nature.

field depends on the value of the ratio (actually contained in the S-function). If we now imagine - aside from our special example - any other ellipse with a much higher main axes ratio we will see that the magnetic field has increased. This would mean that the greater the inclination of the travelled circular trajectory the larger the magnetic field in case of self-interaction, if it is examined under various inclination angles. For now, weleave this result, which definitely is inconsistent with classical experience, to revert to it later.

Now let us start to calculate the energy of the particle. For this purpuse, we use the equations for and

As mentioned above, to specify an energy value only makes sense if there is a magnetic field , i.e. if the magnetic field theorem is satisfied, we must express in such that only the component of that can be recognized in direction 1 will be included into the calculation. That means that 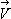must be replaced by in the equation for